Calculations of Margin of Error

[General information about statistics on this page was obtained from http://www.westgroupresearch.com/research/margin.html]

            The following formula was used:

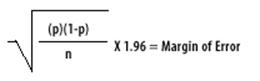
Explanation of the above formula:

1.  ‘p’ is the estimate of the percent of respondents answering a particular question. It is standard industry practice to use 50% (i.e. 0.50) as this is the most conservative. Therefore, p=0.50, 1-p=0.50 and the whole numerator (p)(1-p)=0.25.

2.  The level of precision used is (19 times out of 20), the standard, industry accepted level. This is roughly, 95% (19/20=95%). More precisely, this number represents two standard deviation units, and translates to multiplying by 1.96.

3.  n=sample size

4.  Margin of error is both added and subtracted from the number to produce a range. If the ranges of both numbers, which are compared, overlap, by the rules of statistics, we must conclude that the two numbers are not different - statistically speaking, of course. They must be view as if the difference were produced by a random event – an ‘artifact’ produced by using a sample size that is too small. We could not, therefore, expect that a vote taken by the Community as a whole would, necessarily rule for one side with a majority.

An Example:

Proposition 17f: If Sylvan Lake Public swimming areas became ‘tops optional’, the consequences would be: improved chances of getting laid, reduced chances of getting laid, or no change.

The results:

            Improved chances of getting laid: 61 votes

            No change:                                     51 votes

            Reduced chances of getting laid:     3 votes

Although 113 voters responded to this proposition overall, there are three categories and we want to compare two numbers so there will be three different combinations and each pair will have a different ‘margin of error’, because the number ‘n’ in the formula is the sum of the pair of numbers compared.

Improved Chances vs. Reduced Chances:

            So, comparing the following two categories: ‘Improved changes of getting laid’ and ‘Reduced chances of getting laid’:

            Improved chances of getting laid: 61 votes

            Reduced chances of getting laid:     3 votes

n=64

Using our formula:

Margin of error =  ± the square root of (0.25/64)X1.96=0.122=12.2%

            Improved chances of getting laid: 61 votes ±12.2%= 61 votes ±7.5 votes = a range of 53.5 to 68.5

            Reduced chances of getting laid:     3 votes ±12.2%=3 votes ±0.4 votes= a range of 2.6 votes to 3.4 votes

            Since the two ranges do not overlap, we can conclude, statistically speaking, that the two numbers are different and if a vote were held for the Community as a whole, we would expect that a majority would vote for ‘improved chances of getting laid’, 19 times out of 20 (i.e. if twenty votes were held in a row, which would be a lot of work and therefore, is an excellent reason why we need statistics)

Improved Chances vs. No Change:

            Comparing the following two categories: ‘Improved changes of getting laid’ and ‘No Change’:

            Improved chances of getting laid: 61 votes

            No Change:                                    51 votes

n=112

Using our formula:

Margin of error =  ± the square root of (0.25/112)X1.96=0.122=9.3%

            Improved chances of getting laid: 61 votes±9.3%=61 votes±5.7 votes= a range of 55.3 to 66.7

            No Change:                                    51 votes±9.3%=51 votes±4.7votes= a range of 46.3 to 55.7

            Because the two ranges overlap, the difference between this pair of numbers is not statistically significant. These two numbers could be different only because of the small sample size and a vote of the Community as a whole will not necessarily rule as a majority for either side, 19 times out of 20.

Reduced Chances vs. No Change:

            No change:                                     51 votes

            Reduced chances of getting laid:     3 votes

n=54, therefore using the formula:

Margin of error =  ± the square root of (0.25/54)X1.96=0.122=13.3%

            No change:                                     51 votes±13.3%=51 votes±6.7votes= a range of 44.3 to 57.7

            Reduced chances of getting laid:     3 votes±13.3%=3 votes±0.4 votes= a range of 2.6 to 3.4

            Therefore, the difference between the two numbers is statistically significant.

Summary:

            A majority of Voters, in this survey voted that there would be an improved chances of getting laid if all public swimming areas were tops-optional. The statistical analysis, though, shows that we cannot say, at a 95% confidence level, that such a majority would hold up if the vote were taken by the Community as a whole. We can conclude that it is extremely likely that the Community, as a whole, do not believe that there would be a reduced chance of getting laid if all public swimming areas were tops-optional. We can also conclude that it is likely that the Community, as a whole, believes that there would be either an increased chance of getting laid or no change if all public swimming areas were tops-optional.

Home  |   Executive Summary  |  Site Map   |  Links